MATH

ヒント：図形の場合には線や同じ角度を書いていくことが大事である．そこからどのように式に持っていくかがポイント．

問題

解答

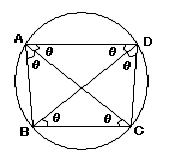
\(∠BAC=\theta\)とおけば\(∠BDC=\theta\)(同位角) また弧において\(AB=BC=CD\)より

\(∠ADB=∠BAC=∠CAD=\theta\)よって\(∠ACD=\pi−3\theta\)

\(\displaystyle \cos(\pi-3\theta)=\frac{9}{16}\Leftrightarrow\cos 3\theta=-\frac{9}{16}\)

ここで\(\cos 3\theta=4\cos^{3}\theta-3\cos\theta\)から

\(4\displaystyle \cos^{3}\theta-3\cos\theta=-\frac{9}{16}\Leftrightarrow 64\cos^{3}\theta-48\cos\theta+9=0\)

ここで予想される因数は\(\displaystyle \pm\frac{9\text{の公約数}}{64\text{の公約数}}\)で当てはめていくと，\(\displaystyle \cos\theta=\frac{3}{4}\)が当てはまる．

∴\((4\cos\theta-3)(16\cos^{2}\theta+12\cos\theta-3)=0\)

∴\(\displaystyle \cos\theta=\frac{3}{4} \frac{-3\pm\sqrt{21}}{8}\)

ここで，\(0<3\theta<\pi\)より \(0<\displaystyle \theta<\frac{\pi}{3}\) ∴ \(\displaystyle \frac{1}{2}<\cos\theta<1\)

この範囲に当てはまるのは\(\displaystyle \cos\theta=\frac{3}{4}\)のみ．

ここで△ABCにおいて，\(AB=a\)とすれば

\(AC=2AB\displaystyle \cos\theta=\frac{3}{2}a\)

∴△ACDで余弦定理より

\(AD^{2}=AC^{2}+CD^{2}-2AC\times CD\cos\angle ACD\) ⇔ \(25=\displaystyle \frac{9}{4}a^{2}+a^{2}-2\times\frac{3}{2}a\times a\times\frac{9}{16}\) ⇔ \(a=4~(a>0)\)

∴\(AC=\displaystyle \frac{3}{2}a=6\)

※三角関数は計算がうまくでき，すぐにが変形できることが重要．(二倍角，加法，半角など)また，和積，積和公式もよくあるから黄チャートや教科書傍用問題集をやって計算力を鍛えるとよい．